Fractal
Introduction
We became interest in fractals as part of our experiments in chaos theory. Music can also be related to chaos theory. Combining music with fractal animation to create music videos just seemed like a classic thing to do.
Remember, chaos is not a messy situation. Chaos is not anarchy. Chaos is the study of unordered systems.
A collection of our classic fractal animation videos can be viewed here.
From Wikipedia, the free encyclopedia
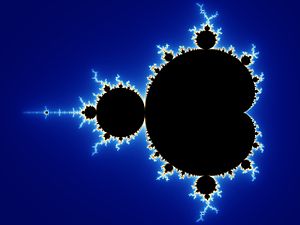
A fractal is generally "a rough or fragmented geometric shape that can be split into parts, each of which is (at least approximately) a reduced-size copy of the whole,"a property called self-similarity. The term was coined by Benoit Mandelbrot in 1975 and was derived from the Latin fractus meaning "broken" or "fractured."
A fractal often has the following features:
- It has a fine structure at arbitrarily small scales.
- It is too irregular to be easily described in traditional Euclidean geometric language.
- It is self-similar (at least approximately or stochastically.)
- It has a Hausdorff dimension which is greater than its topological dimension (although this requirement is not met by space-filling curves such as the Hilbert curve).
- It has a simple and recursive definition.
Because they appear similar at all levels of magnification, fractals are often considered to be infinitely complex (in informal terms). Natural objects that approximate fractals to a degree include clouds, mountain ranges, lightning bolts, coastlines, and snow flakes. However, not all self-similar objects are fractals—for example, the real line (a straight Euclidean line) is formally self-similar but fails to have other fractal characteristics.